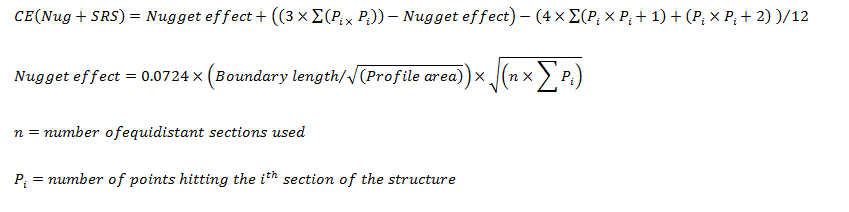Study Review: Dr. Daniel Peruzzi Highlights Best Publication Practices by Analyzing a New Stereological Study
Dr. Daniel Peruzzi, staff scientist, shares his thoughts below:
Customers often ask Staff Scientists at MBF Bioscience why it is sometimes difficult to reproduce certain published stereological results. For example, we get the question, “The estimates that I make of cell number in the region I’m researching do not match numbers reported in the literature. Can you help me understand why?”
To solve this dilemma, we encourage our customers to publish relevant information about their stereological methods to help others reproduce their findings. That’s why it was a pleasure for me to read the recent paper “Postnatal development of the rat amygdala: a stereological study in rats,” by Chareyron, Banta Lavenex, and Lavenex, 2012, reporting stereological estimates of the volume and number of cells contained in the nuclei of the developing rat amygdala.
The authors did an excellent job reporting their stereological methods, including where they researched, and how they performed stereological sampling. As a result, their conclusions are convincing and more easily reproduced by others. I was impressed by both their methods and their findings. In this article I will first summarize their research and then go on to discuss how they reported the particulars of their stereology methods. My main purpose is to point out their stereological reporting and how well thought out their stereologic methods were.
The paper discusses the importance of knowing the anatomical changes that occur in the amygdaloid subdivisions—a place where fear emotions are processed—during development. The amygdaloid complex is involved in all types of emotions, but since bilateral amygdala lesions are rare in humans, most amygdala research regarding fear is done in animals (The Amygdaloid Complex: Anatomy and Physiology, 2003, P. Sah, et.al., Physiol Rev, pp 803-834). Cortical, thalamic, hypothalamic, and brainstem information reaches the amygdala and is processed via the extensive interconnections of the sub-nuclei. The targets of the amygdala include cortical, hypothalamic, and brainstem regions. The subdivisions of the amygdaloid complex have roles in receiving, processing, and transmitting. For instance, the basolateral group receives sensory information and the centromedian group is thought to act as an output to the regions that are substrates for the physiological and cognitive components of fear.
The authors have already used stereology to study the development of the monkey amygdala. In this paper they asked a well-defined research question: What are the changes in volume and cell number that happen in rat basolateral and centromedian nuclei from one week to seven months of age? They used the Cavalieri/point counting probe to estimate volume and the Optical Fractionator probe to estimate the number of cells in the lateral, basal, accessory basal, central, and medial nucleus of the amygdala.
The Methods section of a stereology paper should include information about how both the objects and region of interest are defined and how the sampling is done. Chareyron et al.’s Methods has a detailed section, ‘Anatomical boundaries of the amygdala,’ listing references describing the extent of their five regions of interest. They also showed examples in a figure of a cross-section through the regions that were studied in young and old rats. For the objects of interest, there is a paragraph that describes how neurons, oligodendrocytes and astrocytes were distinguished, and a figure that illustrates these criteria. The unbiased design-based sampling scheme is also well described.
According to their paper, they used the Stereo Investigator 9.0 Cavalieri probe with point counting for volume estimates on Nissl-stained sections. The section sub-fraction is given as one-fourth for the amygdaloid nuclei. In other words every fourth section was used, and the starting section was picked randomly. The point spacing however, was not reported. Sections were taken at 40 microns, and this “before histological shrinkage” thickness was used for the Cavalieri estimates. The authors discuss how avoiding the use of the “after histological shrinkage” volume protects the experiment from the vagaries of that artifact.
I asked Dr. Pierre Lavenex about the point spacing for the Cavalieri/point counting on the amygdaloid nuclei and about the Coefficient of Error (CE). He told me they used point spacing of 100 microns for the volume estimates of the nuclei. He also explained that they used the Gunderson CE for these volume estimates. It is similar to the CE used for the number estimates with the Optical Fractionator (see below), but the local term, nugget, is a shape factor:
He also sent me the CE values for all the nuclei, and they are all around 0.05.
The Stereo Investigator 9.0 Optical Fractionator probe was also used to estimate the number of cells in each amygdaloid nucleus. A good part of the methods section, including an entire table, describes sampling parameters. The section sub fraction is the same as for the Cavalieri estimates—one-fourth—except for the medial nucleus where every eighth section was sampled. The area sub-fractions are also given.
Once the sections are on the microscope, we know what percentage was sampled in the XY plane because the authors provide the size of the counting frames and the size of the grid step between counting frames. Regarding height of the sections, it’s important to have enough room to have several focal planes through the disector. The authors note the use of a 100X Plan Fluor Oil objective with a numerical aperture of 1.3, which gives them a z-focal plane that is a fraction of a micron. The average section thickness and range of section thicknesses is also supplied, as well as the guard zone height and the disector height. They even tell us that section thickness was measured at every counting site instead of just once or twice per section. This gives a better section height and therefore a better height-fraction and estimate. The disector heights were three or four microns. While on the short side, z-planes of one-half micron (a conservative estimate, the z-plane thickness is probably less than one-half micron) yield six to eight focal planes here. That should be enough to make good decisions about whether the top of the cell or another unique point being used to count the cell is in the disector. The authors did not note if they are using the cell-top, the nucleus top, or the nucleolus (only valid if there is one and only one nucleolus per cell) when counting. I assume they were using one of these since they are diligent about all the other rules that eliminate bias. The range and the average number of cells counted per nucleus are also given, which gives us a chance to see the raw counts used for extrapolation based on the volume fraction.
I asked Dr. Lavenex if they used a unique point to count cells, and why they picked the guard zones that they did. My assumption was right; they were correctly using a unique point: “A neuron was counted when the nucleolus of that neuron came into focus. A glial cell was counted when the nucleus of that glia cell came into focus,” Dr. Lavenex said.
As far as guard zones, here are the criteria: “The appropriate guard zone was determined empirically by making different trials with different values from 1 to 2 µm. We found that the value of 1 µm induced bias in comparison with observations made with 1.5 and 2 µm. We were also interested in comparing our data obtained in rats with monkey data obtained in a previous study, and decided to keep the same guard zone (i.e., 2 µm) for animals older than 1 week. For 1 week old rats, for which the tissue shrinkage is maximum (40 µm sections shrink to 6 µm), we chose a guard zone thickness of 1.5 µm to have a sufficient disector height (i.e., 3 µm).”
The authors not only do a great job laying out the sampling parameters, but they also tell us why these sampling intervals were picked—the sampling described drives the coefficient of error (CE) down, close to one-tenth, indicating that the number of cells counted per section is similar from section to section.
I know this because they indicate what CE they are using for the optical fractionator number estimates. The CE they use has two terms that are added together. The first term is the familiar quadratic-based Gunderson CE, which is the part that compares the number of cells from section to section, and drives the CE higher the more variability encountered. I’m not familiar with the second term—the coefficient of variation of the thickness. This is the standard deviation of the section thickness divided by the mean section thickness. This means the more variability there is in thickness compared to the mean thickness, the higher the CE is. I think it is trying to address problems that could come up with the height fraction. If the height varies greatly compared to the mean height, then the height fraction could be suspect. But isn’t the same data that goes into this CE term also the data that is used to calculate the ‘average mounted thickness,’ thus providing a height fraction that already takes into account section-height variability? When I asked Dr. Lavenex about whether this term is too conservative he replied:
“The coefficient of error used for neuron counting takes into account thickness variability. It is important to integrate this factor in the calculation of the coefficient of error because the estimates of the number of cells are directly proportional to the thickness. Effectively, to obtain cell number estimates, the height of the dissector relative to the thickness of the section enters into the calculations. Estimates are thus highly dependent upon the accuracy of the measure of section thickness. That is why the thickness coefficient of error must be added to the counting coefficient of error.”
All of this information in their Methods section allows us to know the what, where, and how of sampling. I’m glad the authors reported the number and volume estimates separately and not as a density, so we can see what is going on behind the density changes; density is affected by both size and number changes. We always encourage researchers to report on them separately, just as these authors did.
Differences in development of the amygdaloid nuclei that could be associated with the emergence of different types of fear behavior are shown. As far as number of neurons, no developmental changes were detected in four of the nuclei. In the basal nucleus, however, there was a statistically significant change in the number of cells. The authors don’t trust this though, because when they do the same ‘deviation from the mean statistics’ on the total number counted in the amygdala (the sum of the five nuclei) there is no significance. I asked Dr. Lavenex to comment on this:
As detailed in the result section of the article, although we observed differences in the number of neurons in the basal nucleus, there was no statistical difference in neuron number among 1-week-old, 3-week-old, 4-week-old, and 7-month-old rats in this nucleus. The difference observed suggested that neuron number would decrease after 1 week, increase after 2 weeks, and then decrease again until 2 months to eventually increase to reach 1-week level at 7 month what is far from probable. That is why we qualified this difference “spurious.” This difference could possibly be explained by little variations in the delineation of the different amygdala nuclei that is congruent with the observation that this difference disappeared when we added together the number of neurons in all nuclei.
In each nucleus, the number of astrocytes after two months of age was less than the number before three weeks of age. In contrast, the number of oligodendrocytes went up in each nucleus. Since the volume of the nuclei went up during the first three weeks of life but the number of cells did not, the authors attribute the volume increase to increases in the neuropil. Increases in volume after three weeks of age correlate with increases in the number of oligodendrocytes and may be due to increased myelination of fibers within the amygdala. Regarding developmental size changes, the volume of the centromedian nuclei is better established at birth than the volume of the basolateral nuclei. The latter nuclei have a lot more post-natal growing to do. The authors speculate that the early maturation of the central nucleus could be involved with the early emergence of simple fear reactions, such as fleeing and freezing, and the correlation of the later development of the basolateral group with the later expression of more complex fear behaviors may also indicate a cause and effect relationship.
Due to the authors’ thorough work in this paper, the readers are in an excellent position to speculate right along with the authors, since all of the important details about the parameters of the research are thoroughly documented.
My thanks to the corresponding author, Dr. Lavenex, for promptly providing additional information for this article.
Chareyron, L. J., Lavenex, P. B. and Lavenex, P. (2012), Postnatal development of the amygdala: A stereological study in rats. J. Comp. Neurol., 520: 3745–3763. doi: 10.1002/cne.23132